Photo Electric Effect : Definition, Types and Everything

When the radiations fall on any matter, it may cause the separation of electrons from the atoms so that an electric current may be caused to flow. This is called the photoelectric effect. It can be produced by radiations of all wavelengths (From the shortest cosmic rays to the longest infrared rays). The fundamental laws that govern the photoelectric phenomenon are the same for all wavelengths.

The photoelectric effect, involving the release of electrons from matter by light, fall into the following three main groups:

1. Surface photoelectric effect
2. Volume photoelectric effect
3. Photovoltaic effect

1. Surface photoclectric effect

The term surface photoelectric effect (or simply photoelectric effect) is used for the release of electrons from a solid when the light falls on it. It usually implies the ejection of photoelectrons from the metal’s surface.

2. Volume photoelectric effect

The volume photo-electric effect involves the release of electrons throughout a finite volume under the influence of light. It is observed that there is a change in the conductivity of the material due to the volume photo-electric effect. The effect is referred to as photo- conductivity. The volume photo-electric effect cannot be detected in a metal because of its high natural conductivity.

3. Photovoltaic effect

The term photovoltaic effect refers to the generation of an electro- motive force in a circuit containing the sensitive material under the influence of light, so that a current may flow without the presence of a battery in the circuit.

Discovery of photoelectric effect

Photoelectric effect was first observed by Hertz in 1887. He found that a spark would jump a greater distance between two electrodes of a circuit when ultraviolet light was falling on the electrodes than when it was not falling. Some simple experiments performed by him led him to even suspect that the discharge was initiated by some process taking place at the negative electrode.

Hallwachs in 1888 found that an insulated uncharged polished zinc plate when illuminated by ultraviolet light acquired positive charge as indicated by an electro-scope. If the plate had negative charge, the charge began to leak as soon as light was turned on, but if it had strong positive charge the incident light had no effect on the charge. To explain this behaviour, Hallwachs suggested that the incident light caused the emission of negatively charged particles from the zinc plate and that when the plate had a strong positive charge, these particles were prevented from escaping from the plate.

Lenard and Thomson showed in 1899 that the charged particles released from the metal surfaces by light were simply electrons. These are known as photo-electrons.

Experimental study of photoelectric effect

The phenomenon of photoelectric effect can be studied using the arrangement shown in Fig.

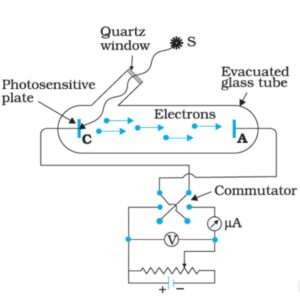
A sensitive plate K, (say of zinc) is enclosed in an evacuated glass envelope with a quartz window (quartz is used for the window as it is transparent to visible as well as ultraviolet light). A is a wire sealed through the wall of the envelope and serves as a collecting electrode. A potential divider is connected across K and A in such a manner that the potential of K with respect to A can be varied continuously from a negative value to a positive value of a few volts. When the light is allowed to fall on K electrons are liberated from its surface; these are attracted and collected by the electrode A and move back to K via the external circuit. Thus, a photo-current is observed in the sensitive galvanometer G.

If the intensity of the incident light is varied, the photo-electric current is found to vary in direct proportion to it. When the intensity of light is kept constant and the potential difference between K and A is decreased making K less negative with respect to A, the photo-current first remains almost constant. This constant value of the current is known as saturation current. And then starts decreasing and finally becomes zero for a certain potential difference between the two electrodes.

(See the graph given below).

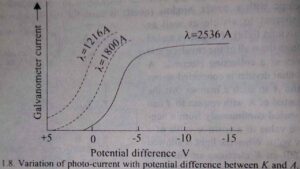
The current does not fall to zero when the two electrodes are at the same potential or even when K is slightly positive with respect to A. This is due to the fact that when K is positive with respect to A, the electrons emitted from it are attracted back towards it and in order that an electrons may reach A it will have to do a certain amount of work equal to eV against this force of attraction, where is the potential difference between K and A.

If an electron possesses a kinetic energy 1/2(mv²) ≥ eV

it can reach A and contribute to the current through the galvanometer. Here m is the mass and e is the charge of an electron and v is its velocity. Thus, for a critical value V_s of the potential difference between K and A no electron will be able to reach A and the photo-current will be zero. This potential of K with respect to A is known as stopping potential and is given by the equation

E_max = 1/2(mv²_max) =eV_s —- (1)

where E_max is the maximum kinetic energy and v_max is the corresponding maximum velocity. Here v_max is that velocity with which the photo-electrons can be ejected from the surface under the given conditions. The stopping potential and therefore the maximum kinetic energy E_max is found to be independent of the intensity of light, i.e., it is a characteristic of the metal surface from which the electrons are ejected. The saturation current is however found to increase in proportion to the intensity of light.

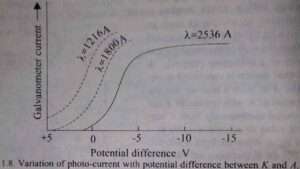
If, next, the frequency of the incident light is varied it is found that the stopping potential, although constant for a given frequency, increases with increasing frequency. The graphs corresponding to three wavelengths are given in the above figure. The graph drawn in Fig. given below is the plot of the maximum kinetic energy of the photo-electron. Which is proportional to V_s against frequency ν, for three different metals.

See the graph

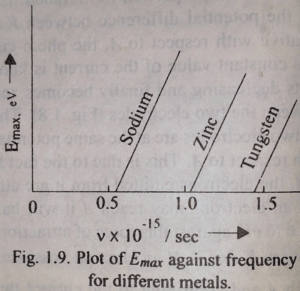
The graph shows that there is always a linear relationship between the maximum kinetic energy and fre- quency v. This linear relationship can be expressed as

E_max = eV_s = hν – W — (2)

where h and W are constants.

It is found from the graph and also from eqn. (2) that for a certain W critical frequency of the incident light

ν_o = W/h

the maximum kinetic energy E_max of the photo-electron is zero. This minimum frequency is known as threshold frequency of the photo-electric effect. This is the limiting frequency below which no photo-electric effect can occur. Threshold frequency depends upon the nature of the target metal as is seen from the graph. The eqn. (2) can also be written as, putting the value of W in terms of ν_o,

E_max = hν – hν_o —-(3)

Laws of photo-electricity

The study of the phenomenon of photoelectric effect leads to the following three fundamental laws of photo electricity.

1. The number of photo-electrons released per second or the photo-electric current is directly proportional to the intensity of the incident light.
2. The energy with which the photo-electron is liberated is a linear function of the frequency of light responsible for liberating it.
3. There is no effect of the intensity of light on The energy of the photoelectrons.

Einstein’s explanation of the photo-electric effect

At the first glance the electromagnetic theory of light seems to be capable of explaining the photo-clectric effect. The electric and magnetic fields which are attributed to light waves in this theory, may well be able to exert sufficient force on the electrons in the metal and release them from its surface. However on this basis it should be expected that the light of higher intensity i.e., consisting of stronger fields would give higher kinetic energy to the electrons liberated from the surface in contradiction to the observed results. It would further be expected that light of low frequency (infrared) if sufficiently intense would be as much effective as the light of high frequency (ultra-violet), which is again in contradiction to the experiments which show that light of frequency below a sharply defined threshold is absolutely incapable of producing any effect.

Thus, it is concluded that the electromagnetic theory completely fails to explain the photoelectric effect. Remamber wave theory could so successfully explain other phenomenon exhibited by light, such as interference and diffraction,

A satisfactory explanation of photoelectric effect was given by Einstein. For this he made the assumption which Planck had to make earlier, that the emission of radiation takes place in little bursts or packets known as photon or quanta rather than continuously. The energy (E) of each quantum is represented by:

E = hν

where h is known as Planck’s constant and is the same as that used in eqn. (3). Hence, individual photons have smaller energy content for light of smaller frequency and a larger one for light of higher frequency. Einstein further accepted that light is not only emitted in quanta but it also travels as quanta. He also postulated that each photon or quantum of energy hν can be transferred as a whole to an electron in a metal. When a photon strikes, a metal surface, its energy hν is used in two parts. One part W being used for the liberation of an electron from the metal. The other part being used for giving kinetic energy 1/2(mv²_max) to the electron :

E = hν =W+1/2(mv²_max) ……. (4)

This is known as Einstein’s photoelectric equation and is the same as eqn. (2). Here, W is work function of the given metal surface. This work function is equal to the minimum energy hν that the photon must have, so that it may just dislodge an electron from the metal surface. In other words W is the work that the electron has to do in order that it may just escape from the surface. The eqn. (4) can therefore be written as

hν = hν_o + 1/2(mv²_max) ———– (5)

The theory therefore clearly predicts a threshold frequency v below which no photo-electric effect will be possible.

The electrons coming from a small depth within the metal have to make their way through several atoms before reaching the surface and therefore lose a part of their energy. They would obviously emerge from the surface with smaller energics than the electrons which come just beneath the surface. It is because of this fact that all the electrons do not emerge from the surface with the same energy but with all energies upto a value E_max = 1/2(mv²_max) as observed experimentally.

In Einstein’s quantum theory, light of high intensity is not described as due to very energetic photon but instead to a very large number of photons per second travelling as a ray of light. Thus, light of higher intensity is expected to produce greater number of photo-electrons per second. It is causing a larger photo-electric current without however giving to each electron a greater energy than does the light of lower intensity and of the same frequency. This agrees with the observed results.

Also Read :

To Wikipedia : Click Here

Thanks for visit and read the topic photoclectric effect…